動機
Hard題都是來看創意的
把 group encode成起點與終點
同時這題也是遇過最複雜的一題
Problem
There are n items each belonging to zero or one of m groups where group[i] is the group that the i-th item belongs to and it's equal to -1 if the i-th item belongs to no group. The items and the groups are zero indexed. A group can have no item belonging to it.
Return a sorted list of the items such that:
- The items that belong to the same group are next to each other in the sorted list.
- There are some relations between these items where
beforeItems[i]is a list containing all the items that should come before thei-th item in the sorted array (to the left of thei-th item).
Return any solution if there is more than one solution and return an empty list if there is no solution.
Example 1:
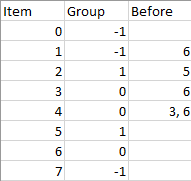
Input: n = 8, m = 2, group = [-1,-1,1,0,0,1,0,-1], beforeItems = [[],[6],[5],[6],[3,6],[],[],[]]Output: [6,3,4,1,5,2,0,7]
Example 2:
Input: n = 8, m = 2, group = [-1,-1,1,0,0,1,0,-1], beforeItems = [[],[6],[5],[6],[3],[],[4],[]]Output: []Explanation: This is the same as example 1 except that 4 needs to be before 6 in the sorted list.
Constraints:
1 <= m <= n <= 3 * 104group.length == beforeItems.length == n-1 <= group[i] <= m - 10 <= beforeItems[i].length <= n - 10 <= beforeItems[i][j] <= n - 1i != beforeItems[i][j]beforeItems[i]does not contain duplicates elements.
Sol
n+ group_idx是group的起點
n++m group_idx是group的終點
接著從終點開始走回去(因為是dfs),所以從數字大的(group的終點)走回去
class Solution:
def sortItems(self, n: int, m: int, group: List[int], beforeItems: List[List[int]]) -> List[int]:
prog = defaultdict(int) # 1 process, 2 done
gh = defaultdict(set)
not_used = set(range(n))
# add group
for (i,g) in enumerate(group):
if g != -1:
gh[n+g].add(i) # entry of group
gh[i].add(n+m+g) # exit of group
# add before relation
for (i,bs) in enumerate(beforeItems):
for b in bs:
if group[i] == group[b] and group[i] != -1:
gh[b].add(i)
else:
i_entry = n+group[i] if group[i] != -1 else i
b_exit = n+m+group[b] if group[b] != -1 else b
gh[b_exit].add(i_entry)
def topo(i,ret):
if prog[i] == 1:
raise 'loop'
elif prog[i] == 2:
return []
else:
prog[i] = 1
[topo(x,ret) for x in gh[i]]
if i<n:
not_used.remove(i)
ret.append(i)
prog[i] = 2
return ret
try:
order = sorted(gh.keys())[::-1]
dep = []
[topo(i,dep) for i in order]
return dep[::-1]+list(not_used)
except:
return []